The hardware and bandwidth for this mirror is donated by METANET, the Webhosting and Full Service-Cloud Provider.
If you wish to report a bug, or if you are interested in having us mirror your free-software or open-source project, please feel free to contact us at mirror[@]metanet.ch.

DoseFinding

CRAN status

The DoseFinding package provides functions for the design and analysis of dose-finding experiments (for example pharmaceutical Phase II clinical trials). It provides functions for: multiple contrast tests, fitting non-linear dose-response models, a combination of testing and dose-response modelling and calculating optimal designs, both for normal and general response variable.

Installation

You can install the development version of DoseFinding from GitHub with:

# install.packages("devtools")
devtools::install_github("bbnkmp/DoseFinding")

Examples

Performing multiple contrast tests

library(DoseFinding)
data(IBScovars)

## set random seed to ensure reproducible adj. p-values for multiple contrast test
set.seed(12)

## perform (model based) multiple contrast test
## define candidate dose-response shapes
models <- Mods(linear = NULL, emax = 0.2, quadratic = -0.17,
               doses = c(0, 1, 2, 3, 4))
## plot models
plot(models)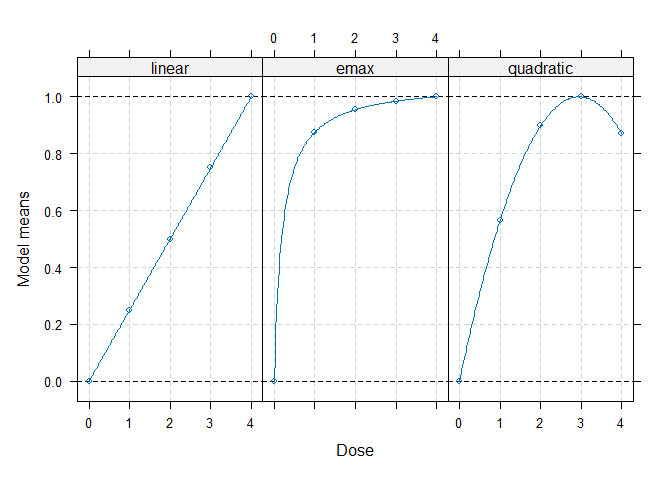

## perform multiple contrast test
MCTtest(dose, resp, IBScovars, models=models,
                addCovars = ~ gender)
#> Multiple Contrast Test
#> 
#> Contrasts:
#>   linear   emax quadratic
#> 0 -0.616 -0.889    -0.815
#> 1 -0.338  0.135    -0.140
#> 2  0.002  0.226     0.294
#> 3  0.315  0.252     0.407
#> 4  0.638  0.276     0.254
#> 
#> Contrast Correlation:
#>           linear  emax quadratic
#> linear     1.000 0.768     0.843
#> emax       0.768 1.000     0.948
#> quadratic  0.843 0.948     1.000
#> 
#> Multiple Contrast Test:
#>           t-Stat   adj-p
#> emax       3.208 0.00128
#> quadratic  3.083 0.00228
#> linear     2.640 0.00848

Fitting non-linear dose-response model

## fit non-linear emax dose-response model
fitemax <- fitMod(dose, resp, data=IBScovars, model="emax",
                  bnds = c(0.01,5))
## display fitted dose-effect curve
plot(fitemax, CI=TRUE, plotData="meansCI")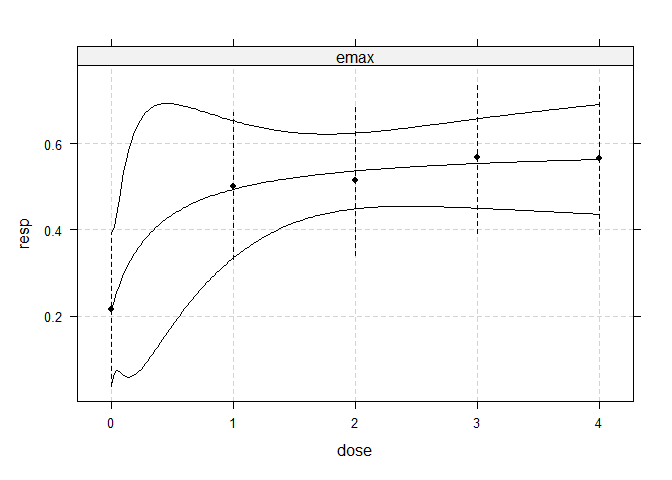

Optimal designs for dose estimation

## Calculate optimal designs for target dose (TD) estimation
doses <- c(0, 10, 25, 50, 100, 150)
fmodels <- Mods(linear = NULL, emax = 25, exponential = 85,
                logistic = c(50, 10.8811),
                doses = doses, placEff=0, maxEff=0.4)
plot(fmodels, plotTD = TRUE, Delta = 0.2)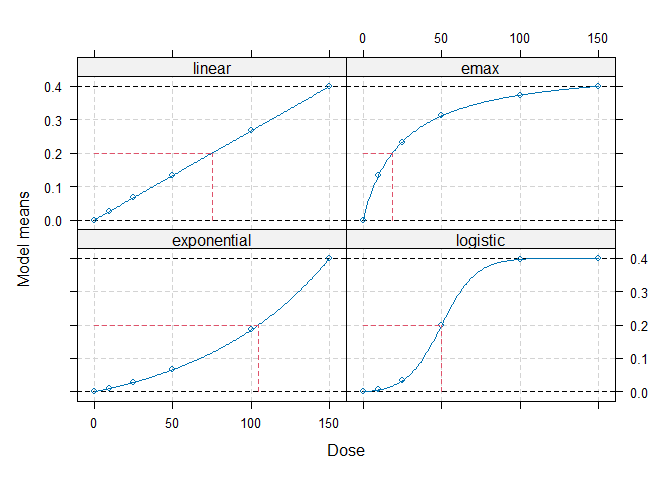

weights <- rep(1/4, 4)
optDesign(fmodels, weights, Delta=0.2, designCrit="TD")
#> Calculated TD - optimal design:
#>       0      10      25      50     100     150 
#> 0.34960 0.09252 0.00366 0.26760 0.13342 0.15319

These binaries (installable software) and packages are in development.
They may not be fully stable and should be used with caution. We make no claims about them.